「ガラパゴ三角関数」の版間の差分
| (同じ利用者による、間の38版が非表示) | |||
| 1行目: | 1行目: | ||
| − | '''ガラパゴ三角関数'''(ガラパゴさんかくかんすう)とは、$$+1$$ と $$z=e^{i\theta}$$ を理論上の基底の元($$z$$ | + | '''ガラパゴ三角関数'''(ガラパゴさんかくかんすう)とは、$$+1$$ と $$z=e^{i\theta}$$ を理論上の基底の元($$z$$ が実数であっても独立した元であるものとみなして区別)とする斜交座標系において、極座標 $$e^{xz}$$ を基底の元の線形結合で表現したときの各元の係数を得る関数である。一般的な三角関数の純粋な拡張として [[みゆ]] により考案された。 |
| − | + | [[ファイル:ガラパゴ三角関数の幾何イメージ.png |480px|center|border|ガラパゴ三角関数の幾何イメージ]] | |
| − | :$$\ | + | |
| − | e^ | + | |
| − | \ | + | ==概要== |
| − | \ | + | ガラパゴ三角関数 $$\cos_zx$$、$$\sin_zx$$ は、次のように表される。 |
| − | \ | + | |
| − | + | ||
| − | + | :$$\begin{align*} | |
| − | + | &\lim_{n\to\infty}\left(1+\frac{x}{n}z\right)^n=e^{xz}=\cos_zx+z\sin_zx\\ | |
| − | + | &\quad\begin{cases} | |
| − | + | z=e^{i\theta}\\ | |
| − | \end{cases} | + | \displaystyle\cos_zx=\lim_{t\to\theta}\left[e^{x\cos t}\cos(x\sin t)-\frac{e^{x\cos t}\sin(x\sin t)}{\tan t}\right]\\ |
| − | + | \displaystyle\sin_zx=\lim_{t\to\theta}\left[\frac{e^{x\cos t}\sin(x\sin t)}{\sin t}\right] | |
| − | + | \end{cases} | |
| − | + | \end{align*}$$ | |
| − | + | ||
| + | この式は | ||
| − | |||
| − | |||
| − | |||
| − | |||
| + | $$\begin{pmatrix}C_{n}&C_{n+1}\\S_{n}&S_{n+1}\end{pmatrix}=\begin{pmatrix}0&-1\\1&2\cos\theta\end{pmatrix}^n$$ あるいは $$\begin{pmatrix}S_{n+1}\\S_{n}\end{pmatrix}=\begin{pmatrix}2\cos\theta&-1\\1&0\end{pmatrix}^n\begin{pmatrix}1\\0\end{pmatrix}$$ より得られる数列 | ||
| − | |||
| − | |||
| + | $$\begin{cases} | ||
| + | S_0=0\\ | ||
| + | S_1=1\\ | ||
| + | S_{n}=-(S_{n-2})+(2\cos\theta)(S_{n-1}) | ||
| + | \end{cases}$$ | ||
| − | |||
| − | + | を用いると、級数展開形にて書き改めることができる。 | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | $$\ | + | :$$\begin{align*} |
| − | + | e^{xz}=&\cos_zx+z\sin_zx\\ | |
| − | + | =&\left(\sum_{k=0}^\infty\frac{C_{k}x^k}{k!}\right)+z\left(\sum_{k=0}^\infty\frac{S_{k}x^k}{k!}\right)\\ | |
| + | =&\left(-\sum_{k=0}^\infty\frac{S_{k-1}x^k}{k!}\right)+z\left(\sum_{k=0}^\infty\frac{S_{k}x^k}{k!}\right)\\ | ||
| + | \end{align*}$$ | ||
| − | $$ | + | ちなみにこの数列 $$S_n$$ は $$z$$ を生成元とする第1種[[ガラパゴ数列]]と同一である。 |
| − | |||
| − | |||
| − | $$\ | + | $$z=e^{i\theta}=\cos\theta+i\sin\theta$$、すなわち $$e^{xz}=e^{x(\cos\theta+i\sin\theta)}=e^{x\cos\theta+ix\sin\theta}$$ であることから、 |
| − | |||
| − | |||
| + | $$e^{xz}$$ の偏角は $$\arg e^{xz}=x\sin\theta$$、絶対値は $$|e^{xz}|=e^{x\cos\theta}$$ であることがわかる。 | ||
| − | |||
| − | |||
| − | |||
| + | $$\{\theta\ne N\pi\mid N\in\mathbb{Z}\}$$ においては $$|e^{xz}|=1$$ へ標準化することで直交座標系との関係性が次のように示される。 | ||
| + | $$\begin{cases} | ||
| + | \alpha=\arg e^{xz}=x\sin\theta\\ | ||
| + | \begin{align*} | ||
| + | e^{i\alpha}=&\cos\alpha+i\sin\alpha\\ | ||
| + | =&e^{-\alpha\cot\theta}\left[\cos_z\frac{\alpha}{\sin\theta}+z\sin_z\frac{\alpha}{\sin\theta}\right]\end{align*} | ||
| + | \end{cases}$$ | ||
| − | + | ==導出== | |
| + | $$z=e^{i\theta}$$ において、 $$e^{xz}=\cos_zx+z\sin_zx$$ の両辺を直交座標形式に変換 | ||
| − | |||
左辺 | 左辺 | ||
| − | \begin{align} | + | :$$\begin{align*} |
e^{xz}=&e^{xe^{i\theta}}\\ | e^{xz}=&e^{xe^{i\theta}}\\ | ||
=&e^{x(\cos\theta+i\sin\theta)}\\ | =&e^{x(\cos\theta+i\sin\theta)}\\ | ||
| 77行目: | 71行目: | ||
=&e^{x\cos\theta}[\cos(x\sin\theta)+i\sin(x\sin\theta)]\\ | =&e^{x\cos\theta}[\cos(x\sin\theta)+i\sin(x\sin\theta)]\\ | ||
=&e^{x\cos\theta}\cos(x\sin\theta)+ie^{x\cos\theta}\sin(x\sin\theta) | =&e^{x\cos\theta}\cos(x\sin\theta)+ie^{x\cos\theta}\sin(x\sin\theta) | ||
| − | \end{align} | + | \end{align*}$$ |
右辺 | 右辺 | ||
| − | \begin{align} | + | :$$\begin{align*} |
| − | &\ | + | &\cos_zx+z\sin_zx\\ |
| − | = | + | =&\cos_zx+(\cos\theta+i\sin\theta)\sin_zx\\ |
| − | = | + | =&\left[\cos_zx+\cos\theta\sin_zx\right]+i\sin\theta\sin_zx |
| − | \ | + | \end{align*}$$ |
| + | |||
| + | |||
| + | 両辺の実部と虚部をそれぞれ比較 | ||
| + | :$$e^{x\cos\theta}\cos(x\sin\theta)=\cos_zx+\cos\theta\sin_zx$$ | ||
| + | :$$e^{x\cos\theta}\sin(x\sin\theta)=\sin\theta\sin_zx$$ | ||
| + | |||
| + | |||
| + | $$\theta$$ が $$\pi$$ の整数倍の場合、 $$1$$ と $$e^{i\theta}$$ は線形従属となってしまうため、$$\theta$$ を $$t$$ とおいて $$t\to\theta$$ の極限として考える | ||
| + | |||
| + | $$\begin{align*} | ||
| + | \cos_zx&=\lim_{t\to\theta}\left[e^{x\cos t}\cos(x\sin t)-\frac{e^{x\cos t}\sin(x\sin t)}{\tan t}\right]\\ | ||
| + | \sin_zx&=\lim_{t\to\theta}\left[\frac{e^{x\cos t}\sin(x\sin t)}{\sin t}\right]\\ | ||
| + | \end{align*}$$ | ||
| + | |||
| + | |||
| + | ==級数展開形== | ||
| + | |||
| + | $$+1$$ と $$z=e^{i\theta}$$ を(理論上の)基底の元とする斜交座標形式の複素数平面において、関数 $$f(x)=e^{xz}$$ を想定する。 | ||
| + | |||
| + | |||
| + | $$\exp$$関数のマクローリン展開より | ||
| + | |||
| + | |||
| + | :$$\displaystyle e^{xz}=\exp(xz)=\sum_{n=0}^{\infty}\frac{(xz)^n}{n!}=\sum_{n=0}^{\infty}\frac{z^n}{n!}x^n$$ | ||
| + | |||
| + | |||
| + | [[ガラパゴ累乗定理]]より、$$z$$ を生成元とする第1種[[ガラパゴ数列]] $$\displaystyle G_n=\frac{z^n-\overline{z}^{~n}}{z-\overline{z}}=\sum_{k=0}^\infty z^{n-2k-1}$$ を用いて | ||
| + | |||
| + | |||
| + | :$$z^n = G_nz-G_{n-1}$$ | ||
| + | |||
| + | |||
| + | と表せるため、 | ||
| + | |||
| + | |||
| + | :$$\begin{align*} | ||
| + | \cos_zx=&-\sum_{k=0}^\infty\frac{G_{k-1}x^k}{k!}\\ | ||
| + | \sin_zx=&+\sum_{k=0}^\infty\frac{G_{k}x^k}{k!}\\ | ||
| + | \end{align*} | ||
| + | $$ | ||
| + | |||
| + | |||
| + | $$z=\mathrm{P}=e^{2\pi i}=1$$ のとき $$\rightarrow~2\cos\theta=2$$ | ||
| + | :$$\displaystyle\cos_zx=(1-x)e^x$$ | ||
| + | :$$\displaystyle\sin_zx=xe^x$$ | ||
| + | |||
| + | |||
| + | $$z=\mathrm{P}^\frac12=e^{\frac{1}{2}\cdotp2\pi i}=-1$$($$1$$ の原始 $$2$$ 乗根)のとき $$\rightarrow~2\cos\theta=-2$$ | ||
| + | :$$\displaystyle\cos_zx=(1+x)e^{-x}$$ | ||
| + | :$$\displaystyle\sin_zx=xe^{-x}$$ | ||
| − | $$\ | + | $$z=\mathrm{P}^\frac13=e^{\frac{1}{3}\cdotp2\pi i}$$($$1$$ の原始 $$3$$ 乗根)のとき $$\rightarrow~2\cos\theta=-1$$ |
| + | :$$\displaystyle\cos_zx=\sum_{k=0}^{\infty}\frac{x^{3k}}{(3k)!}-\sum_{k=0}^{\infty}\frac{x^{3k+2}}{(3k+2)!}$$ | ||
| + | :$$\displaystyle\sin_zx=\sum_{k=0}^{\infty}\frac{x^{3k+1}}{(3k+1)!}-\sum_{k=0}^{\infty}\frac{x^{3k+2}}{(3k+2)!}$$ | ||
| − | + | ||
| − | :$$\ | + | $$z=\mathrm{P}^\frac14=e^{\frac{1}{4}\cdotp2\pi i}$$($$1$$ の原始 $$4$$ 乗根)のとき $$\rightarrow~2\cos\theta=0$$ |
| + | :$$\displaystyle\cos_zx=\sum_{k=0}^{\infty}\frac{x^{4k}}{(4k)!}-\sum_{k=0}^{\infty}\frac{x^{4k+2}}{(4k+2)!}=\cos x$$ | ||
| + | :$$\displaystyle\sin_zx=\sum_{k=0}^{\infty}\frac{x^{4k+1}}{(4k+1)!}-\sum_{k=0}^{\infty}\frac{x^{4k+3}}{(4k+3)!}=\sin x$$ | ||
==exps関数を用いた表現== | ==exps関数を用いた表現== | ||
| − | $$\exp$$ | + | $$\mathrm{exp}_s$$ 関数(skipped exponential 関数)を次のように定義する。 |
| + | |||
| + | $$\displaystyle\exp_s(x)=\left(\sum_{k=0}^\infty\frac{x^{ks}}{(ks)!}\right)$$ | ||
| + | |||
| + | |||
| + | この関数は $$\exp$$ 関数をマクローリン展開した各項のうち、$$x$$ の指数が $$s$$ の倍数である項のみによって構成される関数であり、$$s$$ 階微分することで元の関数と一致する('''周階導関数''')。 | ||
| + | |||
| + | |||
| + | この関数を $$m$$ 階微分すると | ||
| + | |||
| + | $$\begin{align*} | ||
| + | \mathrm{exp}^{(m)}_s(x) | ||
| + | =&\frac{1}{s}\sum_{k=0}^{s-1}\left[\left(e^{\frac{m}{s}\cdot2\pi i}\right)^ke^{\left(e^{\frac{2\pi i}{s}}\right)^kx}\right]\\ | ||
| + | =&\frac{1}{s}\sum_{k=0}^{s-1}\left[\exp\left(\frac{2km\pi}{s}i+\exp\left(\frac{2k\pi}{s}i\right)x\right)\right] | ||
| + | \end{align*}$$ | ||
| + | |||
| + | と表されるが、マクローリン展開形を考えれば単に各項の次数が落ちるだけと見ることができる。 | ||
| + | |||
| + | すなわち、$$m=0$$ から $$m=s-1$$ までの $$s$$ 種類の関数を標準基底の元とすることで、あらゆる $$s$$ 階の周階導関数を構成可能である。 | ||
| + | |||
| + | |||
| + | ガラパゴ三角関数は $$z=e^{i\theta}$$ が実数ではなく、かつ、$$\frac{\theta}{2\pi}$$ が有理数のときに周階導関数となるため、$$\mathrm{exp}_s$$ 関数の $$m$$ 階導関数を用いて以下のように示すことが可能である。 | ||
| + | |||
| + | |||
| + | $$z=\mathrm{P}^{\frac13}=e^{\frac{2\pi}3i}$$ の場合 | ||
| + | \begin{array}{c} | ||
| + | +\cos_{z}x &=& \exp_3^{(0)}x-\exp_3^{(1)}x &\leftarrow[+1,~~0~,-1]\\ | ||
| + | -\sin_{z}x &=& \exp_3^{(1)}x-\exp_3^{(2)}x &\leftarrow[~~0~,-1,+1]\\ | ||
| + | -(\sin_{z}x)' &=& \exp_3^{(2)}x-\exp_3^{(0)}x &\leftarrow[-1,+1,~~0~]\\ | ||
| + | \\ | ||
| + | -\cos_{z}x &=& -\exp_3^{(0)}x+\exp_3^{(1)}x &\leftarrow[-1,~~0~,+1]\\ | ||
| + | +\sin_{z}x &=& -\exp_3^{(1)}x+\exp_3^{(2)}x &\leftarrow[~~0~,+1,-1]\\ | ||
| + | +(\sin_{z}x)' &=& -\exp_3^{(2)}x+\exp_3^{(0)}x &\leftarrow[+1,-1,~~0~]\\ | ||
| + | \end{array} | ||
| + | |||
| + | |||
| + | $$z=\mathrm{P}^{\frac14}=e^{\frac{2\pi}4i}=e^{\frac{\pi}2i}$$ の場合 | ||
| + | \begin{array}{c} | ||
| + | +\cos x &=& +\cos_zx &=& \exp_4^{(0)}x-\exp_4^{(2)}x &\leftarrow[+1,~~0~,-1,~~0~]\\ | ||
| + | -\sin x &=& -\sin_zx &=& \exp_4^{(1)}x-\exp_4^{(3)}x &\leftarrow[~~0~,-1,~~0~,+1]\\ | ||
| + | -\cos x &=& -\cos_zx &=& \exp_4^{(2)}x-\exp_4^{(0)}x &\leftarrow[-1,~~0~,+1,~~0~]\\ | ||
| + | +\sin x &=& +\sin_zx &=& \exp_4^{(3)}x-\exp_4^{(1)}x &\leftarrow[~~0~,+1,~~0~,-1]\\ | ||
| + | \end{array} | ||
| + | |||
| + | |||
| − | \begin{ | + | $$z=\mathrm{P}^{\frac15}=e^{\frac{2\pi}5i}$$ の場合($$\phi'=\phi^{-1}=\frac{\sqrt5-1}2$$) |
| − | \ | + | \begin{array}{c} |
| − | =&\ | + | +\cos_zx &=& +\exp_5^{(0)}x-\exp_5^{(3)}x-\phi'\exp_5^{(2)}x+\phi'\exp_5^{(1)}x &\leftarrow[+1~,~~0~~,-1~,-\phi',+\phi']\\ |
| − | =&\ | + | -\sin_zx &=& +\exp_5^{(1)}x-\exp_5^{(4)}x-\phi'\exp_5^{(3)}x+\phi'\exp_5^{(2)}x &\leftarrow[~~0~~,-1~,-\phi',+\phi',+1~]\\ |
| − | =&\ | + | -(\sin_zx)' &=& +\exp_5^{(2)}x-\exp_5^{(5)}x-\phi'\exp_5^{(4)}x+\phi'\exp_5^{(3)}x &\leftarrow[-1~,-\phi',+\phi',+1~,~~0~~]\\ |
| − | \end{ | + | -(\sin_zx)'' &=& +\exp_5^{(3)}x-\exp_5^{(0)}x-\phi'\exp_5^{(5)}x+\phi'\exp_5^{(4)}x &\leftarrow[-\phi',+\phi',+1~,~~0~~,-1~]\\ |
| + | -(\sin_zx)''' &=& +\exp_5^{(4)}x-\exp_5^{(1)}x-\phi'\exp_5^{(0)}x+\phi'\exp_5^{(5)}x &\leftarrow[+\phi',+1~,~~0~~,-1~,-\phi']\\ | ||
| + | \\ | ||
| + | -\cos_zx &=& -\exp_5^{(0)}x+\exp_5^{(3)}x+\phi'\exp_5^{(2)}x-\phi'\exp_5^{(1)}x &\leftarrow[-1~,~~0~~,+1~,+\phi',-\phi']\\ | ||
| + | +\sin_zx &=& -\exp_5^{(1)}x+\exp_5^{(4)}x+\phi'\exp_5^{(3)}x-\phi'\exp_5^{(2)}x &\leftarrow[~~0~~,+1~,+\phi',-\phi',-1~]\\ | ||
| + | +(\sin_zx)' &=& -\exp_5^{(2)}x+\exp_5^{(0)}x+\phi'\exp_5^{(4)}x-\phi'\exp_5^{(3)}x &\leftarrow[+1~,+\phi',-\phi',-1~,~~0~~]\\ | ||
| + | +(\sin_zx)'' &=& -\exp_5^{(3)}x+\exp_5^{(1)}x+\phi'\exp_5^{(0)}x-\phi'\exp_5^{(4)}x &\leftarrow[+\phi',-\phi',-1~,~~0~~,+1~]\\ | ||
| + | +(\sin_zx)''' &=& -\exp_5^{(4)}x+\exp_5^{(2)}x+\phi'\exp_5^{(1)}x-\phi'\exp_5^{(0)}x &\leftarrow[-\phi',-1~,~~0~~,+1~,+\phi']\\ | ||
| + | \end{array} | ||
| − | |||
| − | \ | + | $$z=\mathrm{P}^{\frac16}=e^{\frac{2\pi}6i}=e^{\frac{\pi}3i}$$ の場合 |
| − | + | \begin{array}{c} | |
| − | + | +\cos_{z}x &=& \exp_6^{(0)}x-\exp_6^{(4)}x-\exp_6^{(3)}x+\exp_6^{(1)}x &\leftarrow[+1,~~0~,-1,-1,~~0~,+1]\\ | |
| − | \ | + | -\sin_{z}x &=& \exp_6^{(1)}x-\exp_6^{(5)}x-\exp_6^{(4)}x+\exp_6^{(2)}x &\leftarrow[~~0~,-1,-1,~~0~,+1,+1]\\ |
| − | + | -(\sin_{z}x)' &=& \exp_6^{(2)}x-\exp_6^{(0)}x-\exp_6^{(5)}x+\exp_6^{(3)}x &\leftarrow[-1,-1,~~0~,+1,+1,~~0~]\\ | |
| − | + | \\ | |
| − | + | -\cos_{z}x &=& \exp_6^{(3)}x-\exp_6^{(1)}x-\exp_6^{(0)}x+\exp_6^{(4)}x &\leftarrow[-1,~~0~,+1,+1,~~0~,-1]\\ | |
| − | + | +\sin_{z}x &=& \exp_6^{(4)}x-\exp_6^{(2)}x-\exp_6^{(1)}x+\exp_6^{(5)}x &\leftarrow[~~0~,+1,+1,~~0~,-1,-1]\\ | |
| − | + | +(\sin_{z}x)' &=& \exp_6^{(5)}x-\exp_6^{(3)}x-\exp_6^{(2)}x+\exp_6^{(0)}x &\leftarrow[+1,+1,~~0~,-1,-1,~~0~]\\ | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | \ | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
\end{array} | \end{array} | ||
2021年4月6日 (火) 00:42時点における最新版
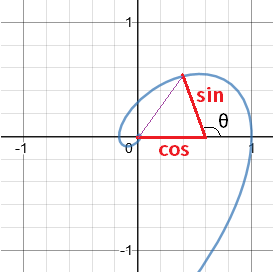
ガラパゴ三角関数(ガラパゴさんかくかんすう)とは、$$+1$$ と $$z=e^{i\theta}$$ を理論上の基底の元($$z$$ が実数であっても独立した元であるものとみなして区別)とする斜交座標系において、極座標 $$e^{xz}$$ を基底の元の線形結合で表現したときの各元の係数を得る関数である。一般的な三角関数の純粋な拡張として みゆ により考案された。
概要
ガラパゴ三角関数 $$\cos_zx$$、$$\sin_zx$$ は、次のように表される。
- $$\begin{align*} &\lim_{n\to\infty}\left(1+\frac{x}{n}z\right)^n=e^{xz}=\cos_zx+z\sin_zx\\ &\quad\begin{cases} z=e^{i\theta}\\ \displaystyle\cos_zx=\lim_{t\to\theta}\left[e^{x\cos t}\cos(x\sin t)-\frac{e^{x\cos t}\sin(x\sin t)}{\tan t}\right]\\ \displaystyle\sin_zx=\lim_{t\to\theta}\left[\frac{e^{x\cos t}\sin(x\sin t)}{\sin t}\right] \end{cases} \end{align*}$$
この式は
$$\begin{pmatrix}C_{n}&C_{n+1}\\S_{n}&S_{n+1}\end{pmatrix}=\begin{pmatrix}0&-1\\1&2\cos\theta\end{pmatrix}^n$$ あるいは $$\begin{pmatrix}S_{n+1}\\S_{n}\end{pmatrix}=\begin{pmatrix}2\cos\theta&-1\\1&0\end{pmatrix}^n\begin{pmatrix}1\\0\end{pmatrix}$$ より得られる数列
$$\begin{cases}
S_0=0\\
S_1=1\\
S_{n}=-(S_{n-2})+(2\cos\theta)(S_{n-1})
\end{cases}$$
を用いると、級数展開形にて書き改めることができる。
- $$\begin{align*} e^{xz}=&\cos_zx+z\sin_zx\\ =&\left(\sum_{k=0}^\infty\frac{C_{k}x^k}{k!}\right)+z\left(\sum_{k=0}^\infty\frac{S_{k}x^k}{k!}\right)\\ =&\left(-\sum_{k=0}^\infty\frac{S_{k-1}x^k}{k!}\right)+z\left(\sum_{k=0}^\infty\frac{S_{k}x^k}{k!}\right)\\ \end{align*}$$
ちなみにこの数列 $$S_n$$ は $$z$$ を生成元とする第1種ガラパゴ数列と同一である。
$$z=e^{i\theta}=\cos\theta+i\sin\theta$$、すなわち $$e^{xz}=e^{x(\cos\theta+i\sin\theta)}=e^{x\cos\theta+ix\sin\theta}$$ であることから、
$$e^{xz}$$ の偏角は $$\arg e^{xz}=x\sin\theta$$、絶対値は $$|e^{xz}|=e^{x\cos\theta}$$ であることがわかる。
$$\{\theta\ne N\pi\mid N\in\mathbb{Z}\}$$ においては $$|e^{xz}|=1$$ へ標準化することで直交座標系との関係性が次のように示される。
$$\begin{cases} \alpha=\arg e^{xz}=x\sin\theta\\ \begin{align*} e^{i\alpha}=&\cos\alpha+i\sin\alpha\\ =&e^{-\alpha\cot\theta}\left[\cos_z\frac{\alpha}{\sin\theta}+z\sin_z\frac{\alpha}{\sin\theta}\right]\end{align*} \end{cases}$$
導出
$$z=e^{i\theta}$$ において、 $$e^{xz}=\cos_zx+z\sin_zx$$ の両辺を直交座標形式に変換
左辺
- $$\begin{align*} e^{xz}=&e^{xe^{i\theta}}\\ =&e^{x(\cos\theta+i\sin\theta)}\\ =&e^{x\cos\theta}\cdot e^{ix\sin\theta}\\ =&e^{x\cos\theta}[\cos(x\sin\theta)+i\sin(x\sin\theta)]\\ =&e^{x\cos\theta}\cos(x\sin\theta)+ie^{x\cos\theta}\sin(x\sin\theta) \end{align*}$$
右辺
- $$\begin{align*} &\cos_zx+z\sin_zx\\ =&\cos_zx+(\cos\theta+i\sin\theta)\sin_zx\\ =&\left[\cos_zx+\cos\theta\sin_zx\right]+i\sin\theta\sin_zx \end{align*}$$
両辺の実部と虚部をそれぞれ比較
- $$e^{x\cos\theta}\cos(x\sin\theta)=\cos_zx+\cos\theta\sin_zx$$
- $$e^{x\cos\theta}\sin(x\sin\theta)=\sin\theta\sin_zx$$
$$\theta$$ が $$\pi$$ の整数倍の場合、 $$1$$ と $$e^{i\theta}$$ は線形従属となってしまうため、$$\theta$$ を $$t$$ とおいて $$t\to\theta$$ の極限として考える
$$\begin{align*} \cos_zx&=\lim_{t\to\theta}\left[e^{x\cos t}\cos(x\sin t)-\frac{e^{x\cos t}\sin(x\sin t)}{\tan t}\right]\\ \sin_zx&=\lim_{t\to\theta}\left[\frac{e^{x\cos t}\sin(x\sin t)}{\sin t}\right]\\ \end{align*}$$
級数展開形
$$+1$$ と $$z=e^{i\theta}$$ を(理論上の)基底の元とする斜交座標形式の複素数平面において、関数 $$f(x)=e^{xz}$$ を想定する。
$$\exp$$関数のマクローリン展開より
- $$\displaystyle e^{xz}=\exp(xz)=\sum_{n=0}^{\infty}\frac{(xz)^n}{n!}=\sum_{n=0}^{\infty}\frac{z^n}{n!}x^n$$
ガラパゴ累乗定理より、$$z$$ を生成元とする第1種ガラパゴ数列 $$\displaystyle G_n=\frac{z^n-\overline{z}^{~n}}{z-\overline{z}}=\sum_{k=0}^\infty z^{n-2k-1}$$ を用いて
- $$z^n = G_nz-G_{n-1}$$
と表せるため、
- $$\begin{align*} \cos_zx=&-\sum_{k=0}^\infty\frac{G_{k-1}x^k}{k!}\\ \sin_zx=&+\sum_{k=0}^\infty\frac{G_{k}x^k}{k!}\\ \end{align*} $$
$$z=\mathrm{P}=e^{2\pi i}=1$$ のとき $$\rightarrow~2\cos\theta=2$$
- $$\displaystyle\cos_zx=(1-x)e^x$$
- $$\displaystyle\sin_zx=xe^x$$
$$z=\mathrm{P}^\frac12=e^{\frac{1}{2}\cdotp2\pi i}=-1$$($$1$$ の原始 $$2$$ 乗根)のとき $$\rightarrow~2\cos\theta=-2$$
- $$\displaystyle\cos_zx=(1+x)e^{-x}$$
- $$\displaystyle\sin_zx=xe^{-x}$$
$$z=\mathrm{P}^\frac13=e^{\frac{1}{3}\cdotp2\pi i}$$($$1$$ の原始 $$3$$ 乗根)のとき $$\rightarrow~2\cos\theta=-1$$
- $$\displaystyle\cos_zx=\sum_{k=0}^{\infty}\frac{x^{3k}}{(3k)!}-\sum_{k=0}^{\infty}\frac{x^{3k+2}}{(3k+2)!}$$
- $$\displaystyle\sin_zx=\sum_{k=0}^{\infty}\frac{x^{3k+1}}{(3k+1)!}-\sum_{k=0}^{\infty}\frac{x^{3k+2}}{(3k+2)!}$$
$$z=\mathrm{P}^\frac14=e^{\frac{1}{4}\cdotp2\pi i}$$($$1$$ の原始 $$4$$ 乗根)のとき $$\rightarrow~2\cos\theta=0$$
- $$\displaystyle\cos_zx=\sum_{k=0}^{\infty}\frac{x^{4k}}{(4k)!}-\sum_{k=0}^{\infty}\frac{x^{4k+2}}{(4k+2)!}=\cos x$$
- $$\displaystyle\sin_zx=\sum_{k=0}^{\infty}\frac{x^{4k+1}}{(4k+1)!}-\sum_{k=0}^{\infty}\frac{x^{4k+3}}{(4k+3)!}=\sin x$$
exps関数を用いた表現
$$\mathrm{exp}_s$$ 関数(skipped exponential 関数)を次のように定義する。
$$\displaystyle\exp_s(x)=\left(\sum_{k=0}^\infty\frac{x^{ks}}{(ks)!}\right)$$
この関数は $$\exp$$ 関数をマクローリン展開した各項のうち、$$x$$ の指数が $$s$$ の倍数である項のみによって構成される関数であり、$$s$$ 階微分することで元の関数と一致する(周階導関数)。
この関数を $$m$$ 階微分すると
$$\begin{align*} \mathrm{exp}^{(m)}_s(x) =&\frac{1}{s}\sum_{k=0}^{s-1}\left[\left(e^{\frac{m}{s}\cdot2\pi i}\right)^ke^{\left(e^{\frac{2\pi i}{s}}\right)^kx}\right]\\ =&\frac{1}{s}\sum_{k=0}^{s-1}\left[\exp\left(\frac{2km\pi}{s}i+\exp\left(\frac{2k\pi}{s}i\right)x\right)\right] \end{align*}$$
と表されるが、マクローリン展開形を考えれば単に各項の次数が落ちるだけと見ることができる。
すなわち、$$m=0$$ から $$m=s-1$$ までの $$s$$ 種類の関数を標準基底の元とすることで、あらゆる $$s$$ 階の周階導関数を構成可能である。
ガラパゴ三角関数は $$z=e^{i\theta}$$ が実数ではなく、かつ、$$\frac{\theta}{2\pi}$$ が有理数のときに周階導関数となるため、$$\mathrm{exp}_s$$ 関数の $$m$$ 階導関数を用いて以下のように示すことが可能である。
$$z=\mathrm{P}^{\frac13}=e^{\frac{2\pi}3i}$$ の場合
\begin{array}{c}
+\cos_{z}x &=& \exp_3^{(0)}x-\exp_3^{(1)}x &\leftarrow[+1,~~0~,-1]\\
-\sin_{z}x &=& \exp_3^{(1)}x-\exp_3^{(2)}x &\leftarrow[~~0~,-1,+1]\\
-(\sin_{z}x)' &=& \exp_3^{(2)}x-\exp_3^{(0)}x &\leftarrow[-1,+1,~~0~]\\
\\
-\cos_{z}x &=& -\exp_3^{(0)}x+\exp_3^{(1)}x &\leftarrow[-1,~~0~,+1]\\
+\sin_{z}x &=& -\exp_3^{(1)}x+\exp_3^{(2)}x &\leftarrow[~~0~,+1,-1]\\
+(\sin_{z}x)' &=& -\exp_3^{(2)}x+\exp_3^{(0)}x &\leftarrow[+1,-1,~~0~]\\
\end{array}
$$z=\mathrm{P}^{\frac14}=e^{\frac{2\pi}4i}=e^{\frac{\pi}2i}$$ の場合
\begin{array}{c}
+\cos x &=& +\cos_zx &=& \exp_4^{(0)}x-\exp_4^{(2)}x &\leftarrow[+1,~~0~,-1,~~0~]\\
-\sin x &=& -\sin_zx &=& \exp_4^{(1)}x-\exp_4^{(3)}x &\leftarrow[~~0~,-1,~~0~,+1]\\
-\cos x &=& -\cos_zx &=& \exp_4^{(2)}x-\exp_4^{(0)}x &\leftarrow[-1,~~0~,+1,~~0~]\\
+\sin x &=& +\sin_zx &=& \exp_4^{(3)}x-\exp_4^{(1)}x &\leftarrow[~~0~,+1,~~0~,-1]\\
\end{array}
$$z=\mathrm{P}^{\frac15}=e^{\frac{2\pi}5i}$$ の場合($$\phi'=\phi^{-1}=\frac{\sqrt5-1}2$$) \begin{array}{c} +\cos_zx &=& +\exp_5^{(0)}x-\exp_5^{(3)}x-\phi'\exp_5^{(2)}x+\phi'\exp_5^{(1)}x &\leftarrow[+1~,~~0~~,-1~,-\phi',+\phi']\\ -\sin_zx &=& +\exp_5^{(1)}x-\exp_5^{(4)}x-\phi'\exp_5^{(3)}x+\phi'\exp_5^{(2)}x &\leftarrow[~~0~~,-1~,-\phi',+\phi',+1~]\\ -(\sin_zx)' &=& +\exp_5^{(2)}x-\exp_5^{(5)}x-\phi'\exp_5^{(4)}x+\phi'\exp_5^{(3)}x &\leftarrow[-1~,-\phi',+\phi',+1~,~~0~~]\\ -(\sin_zx)'' &=& +\exp_5^{(3)}x-\exp_5^{(0)}x-\phi'\exp_5^{(5)}x+\phi'\exp_5^{(4)}x &\leftarrow[-\phi',+\phi',+1~,~~0~~,-1~]\\ -(\sin_zx)''' &=& +\exp_5^{(4)}x-\exp_5^{(1)}x-\phi'\exp_5^{(0)}x+\phi'\exp_5^{(5)}x &\leftarrow[+\phi',+1~,~~0~~,-1~,-\phi']\\ \\ -\cos_zx &=& -\exp_5^{(0)}x+\exp_5^{(3)}x+\phi'\exp_5^{(2)}x-\phi'\exp_5^{(1)}x &\leftarrow[-1~,~~0~~,+1~,+\phi',-\phi']\\ +\sin_zx &=& -\exp_5^{(1)}x+\exp_5^{(4)}x+\phi'\exp_5^{(3)}x-\phi'\exp_5^{(2)}x &\leftarrow[~~0~~,+1~,+\phi',-\phi',-1~]\\ +(\sin_zx)' &=& -\exp_5^{(2)}x+\exp_5^{(0)}x+\phi'\exp_5^{(4)}x-\phi'\exp_5^{(3)}x &\leftarrow[+1~,+\phi',-\phi',-1~,~~0~~]\\ +(\sin_zx)'' &=& -\exp_5^{(3)}x+\exp_5^{(1)}x+\phi'\exp_5^{(0)}x-\phi'\exp_5^{(4)}x &\leftarrow[+\phi',-\phi',-1~,~~0~~,+1~]\\ +(\sin_zx)''' &=& -\exp_5^{(4)}x+\exp_5^{(2)}x+\phi'\exp_5^{(1)}x-\phi'\exp_5^{(0)}x &\leftarrow[-\phi',-1~,~~0~~,+1~,+\phi']\\ \end{array}
$$z=\mathrm{P}^{\frac16}=e^{\frac{2\pi}6i}=e^{\frac{\pi}3i}$$ の場合
\begin{array}{c}
+\cos_{z}x &=& \exp_6^{(0)}x-\exp_6^{(4)}x-\exp_6^{(3)}x+\exp_6^{(1)}x &\leftarrow[+1,~~0~,-1,-1,~~0~,+1]\\
-\sin_{z}x &=& \exp_6^{(1)}x-\exp_6^{(5)}x-\exp_6^{(4)}x+\exp_6^{(2)}x &\leftarrow[~~0~,-1,-1,~~0~,+1,+1]\\
-(\sin_{z}x)' &=& \exp_6^{(2)}x-\exp_6^{(0)}x-\exp_6^{(5)}x+\exp_6^{(3)}x &\leftarrow[-1,-1,~~0~,+1,+1,~~0~]\\
\\
-\cos_{z}x &=& \exp_6^{(3)}x-\exp_6^{(1)}x-\exp_6^{(0)}x+\exp_6^{(4)}x &\leftarrow[-1,~~0~,+1,+1,~~0~,-1]\\
+\sin_{z}x &=& \exp_6^{(4)}x-\exp_6^{(2)}x-\exp_6^{(1)}x+\exp_6^{(5)}x &\leftarrow[~~0~,+1,+1,~~0~,-1,-1]\\
+(\sin_{z}x)' &=& \exp_6^{(5)}x-\exp_6^{(3)}x-\exp_6^{(2)}x+\exp_6^{(0)}x &\leftarrow[+1,+1,~~0~,-1,-1,~~0~]\\
\end{array}